How to Determine if a Set Is Countable

By Ra_Katelynn680 18 Apr, 2022 Post a Comment

Advanced Math questions and answers. Determine whether set 𝑆 is countable.

1 11 3 Countable Sets Video Youtube

S N from S to N.

. If it is uncountable prove by contradiction. If it is a finite set mid A mid infty. Determine whether set 𝑆 is countable.

Question 2 Determine if the set of integers divisible by 5 but not divisible by 7 is a countable set. If it is uncountable prove by contradiction. First we prove a.

We will be able to count the elements of an infinite set if we can define a one. Since fA is a subset of the countable set B it is countable and therefore so is A. Thats a union of.

If not briefly explain. Equivalently a set S is countable if there exists an injective function f. All members of the se.

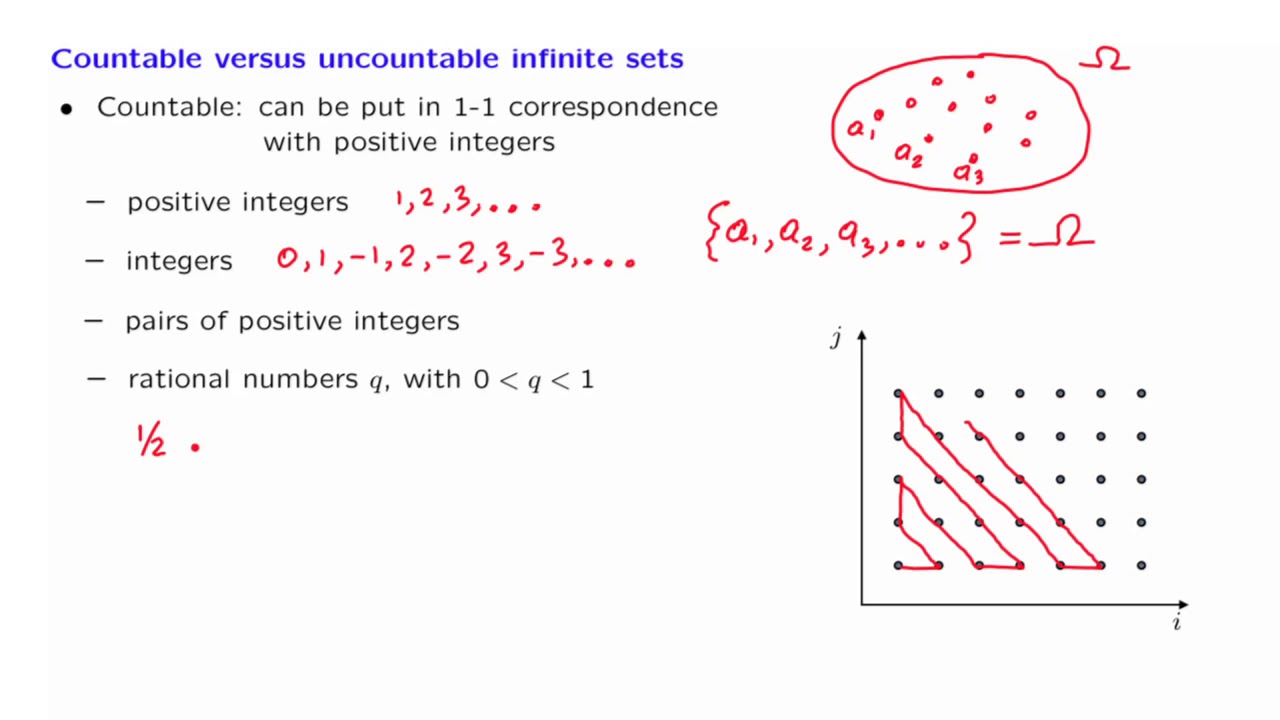
In mathematics a set is countable if it has the same cardinality as some subset of the set of natural numbers N 0 1 2 3. 𝐴0 finite countable or uncountable Cardinality. If you can find a systematic way to do this youll have shown the set is countable.

If the set is countable exhibit the one-to-one correspondence between the set and the set of positive integers. Note that R A T and A is countable. So A is the set of all functions from N Y and so forth.

Select the list of numbers you will count and click Kutools Select Select Specific Cells. Let S be a nonempty set. A countable set is either a finite set or a countably infinite set.

If it is countable find a bijection from 𝑆 to ℤ. Since R is un-countable R is not the union of two countable sets. A fA by setting fx fx for every x A.

𝑆 𝑥 ℝ 10 𝑥. The set of finite languages over any countable alphabet Sigma is the union over all finite SsubsetSigma of the set of finite languages over S. There are only countably many finite subsets of a countable set.

1 S is countable. Suppose B is countable and there exists an injection f. We deﬁne h.

How do I determine which of the above sets is countably infinite uncountably infinite or neither ie. Yet in other words it means you are able to put the elements of the set into a standing line where each one has a waiting number but. Set A is called countable if one of the following is true.

Since A is inﬁnite A is nonempty and so h is well-deﬁned. All members of the set are assigned to different natural numbers and there are no members of the set left over. If it is countable find a bijection from 𝑆 to ℤ.

For example you might do part a by listing the elements as. Start by trying to list all the elements of the set. N A as follows.

The elements of a finite set can be counted by defining a bijection one-to-one correspondence between the set and mathbbN_k for some natural number k. The cardinality size of a set may be determined in various ways depending on the set in question. If the set is infinite being countable means that you are able to put the elements of the set in order just like natural numbers are in order.

Y 1 2 3 n n 3 A from N Y B from R Y C from Y N D from Y R E from Y Y. A B C D and E which are the sets of all functions using the below information. 2 In the Specific type section select the Greater than or equal to from the.

The first step is to get your head around the basic definitions involved. In the opening Select Specific Cells dialog box please see the second screenshot above. 𝑆 𝑥 ℝ 10 𝑥.

So Red Green Blue is a countable set because we can assign 1 to Red 2 to Blue and 3 to Green. 2 There is an injection f. A set is countable if you can count its elements.

Answer 1 of 3. Without loss of generality we may assume that A is an inﬁnite subset of N. For each set determine if it is finite countable or uncountable and find the cardinality.

Let A denote the set of algebraic numbers and let T denote the set of tran-scendental numbers. I have a few sets. If T were countable then R would be the union of two countable sets.

1 Check the Cell option in the Selection type section. Just as in the proof of Theorem 4 on the ﬁnite sets handout we can deﬁne a bijection f. The following three conditions are equivalent.

Having deﬁned hn 1 we deﬁne hn minAh1hn1. We say two sets have the same cardinality when there is a bijection one-to-one matching between thei. It can be put in one-to-one correspondence with natural numbers mathbb N in which case the set is said to be countably infinite.

A useful criterion to determine a set is countable A set is countable if it is either countably in nite or nite. Of course if the set is finite you can easily count its elements. We will show that 1 implies 2 implies 3 implies 1.

Let h1 minA. 3 There is a surjection g. 2 4 6 8 10 is also a countable set because we can 1 to 2 2 to 4 3 to 6 4 to 8 and so on.

Any subset of a countable set is countable. Again since A is inﬁnite the set. It simply means that every element in S corresponds to a different element in N.

Solved Determine If The Sets Are Countable Or Uncountable Chegg Com